Inversion at the 12th in fugue BWV 885

The exposition is followed by a brief episode (measures 18 to 19). In measure 20 the subject is presented by the tenor and the countersubject by the bass:

Another episode leads us to the first inversion at the 12th in measure 28. Note how the tenor - using fragments from the countersubject - occasionally doubles the soprano using thirds:

By examining the resulting intervals we see that Bach is using the inversion at the 12th. We will compare measures 28 and 29 with measures 9 and 10 (this passage is really 4 measures long but for our purposes we will only show the first two measures):

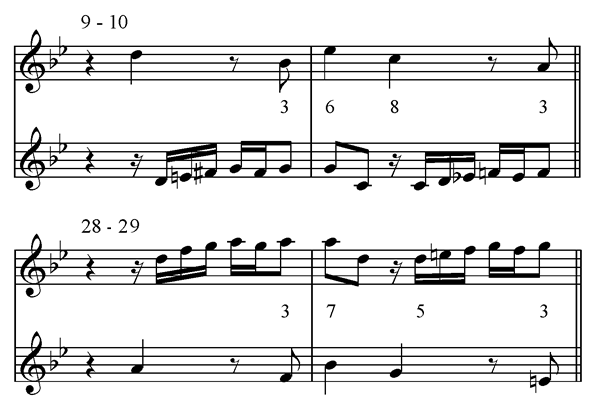
The octaves in measures 9 and 10 become fifths after the inversion. Thirds invert as thirds and the sixths as sevenths. By looking at the inversion at the 12th table we find that the inversions are the result of an inversion at the 12th (click here for a more detailed explanation of invertible counterpoint):

1	2	3	4	5	6	7	8	9	10	11	12
12	11	10	9	8	7	6	5	4	3	2	1

We can also confirm that Bach is using an inversion at the 12th by the fact that the lower voice in measure 10 moves up a ninth in measure 29, while the upper voice moves a fourth. By adding the intervals by which the voices move (4 + 9) and then subtracting 1 ( 4 + 9 = 13, 13 - 1 = 12), we find the type of inversion.